Let’s continue the story in the previous post about Newtonian mechanics.
In Newton’s theory of gravity, two objects that have mass attract each other with a gravitational force simply by virtue of their mass. The strength of the force is proportional to the product of the two masses, and inversely proportional to the square of the distance between the objects. This means that if you move two objects apart, the gravitational force between them will decrease; for example, if you double the distance between the objects, then the force between them will be less by a factor of 1/4.
Newton’s contemporaries (c 1700) asked him to explain how in the world the attraction could take place when the objects were very far apart; for example, the sun and earth are separated by about 150 million kilometres, but nevertheless there is an attractive gravitational force between them. How can this be? One can understand that two colliding objects will exert a force on each other, because they come into contact. How can two distant objects exert a force on each other when they are so very far from touching?
“Hypotheses non fingo,” said Newton, which has been translated as, “I frame no hypotheses.” A different person, with today’s sensibilities, might have said:
Look you fools, I’ve just revolutionized modern science. Isn’t that enough for now? What more do you want from me? Why don’t you go ahead and figure that one out, I think I’ve done enough for several lifetimes.
In other words, I don’t know how two objects separated by astronomical distance can exert a force on each other, and I don’t care to speculate about how, deep down, it happens. I can see the effects, I can calculate the effects, and that ought to be enough.
And it was enough, for a while. Until Michael Faraday came onto the scene, about a century later.
This phenomenon, that two objects can exert a force on each other although separated by great distances, came to be known as “action at a distance.” That is, the phrase “action at a distance” is a convenient summary of the state of affairs back then: “I know it happens, I can calculate it, but I just can’t explain how it happens.”
The lack of a mechanical model for the phenomenon of gravity bothered scientists of the day, but what could they do?
Well, Faraday did do something about this unhappy situation. He invented the field concept, which helped physicists to be a lot more satisfied with their understanding of the phenomenon. And the concept proved so fruitful that it has become the central paradigm in modern physics.
Faraday was motivated by the way iron filings align themselves near a magnet:
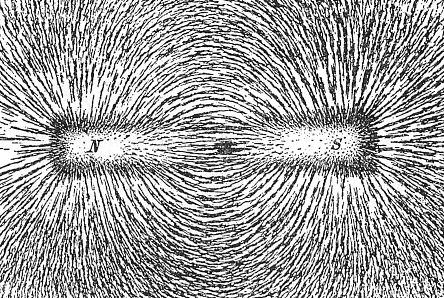
He imagined that the magnet extended some sort of “tentacles” of force throughout the space around it, exerting some sort of tension.This “tension” in the space around the magnet is what causes the iron filings to align.
Might not gravity act in the same sort of way? And electrical forces too? Perhaps all forces arise in the same way: whatever the source of the force is (mass in the case of gravity, electric charge in the case of electric forces, and electric currents in the case of magnetism), the source creates a “tension” of some sort in space. Then an object that is able to respond to the force simply “feels” the “tension” in the “tentacles” at its location.
The collection of tentacles under tension is what we call a field. So the collection of gravitational tentacles is called a gravitational field, the collection of electric tentacles is called an electric field, the collection of magnetic tentacles is called a magnetic field, and so on. Each force has its own type of field.
So this is Faraday’s vision, and the solution to the action-at-a-distance conundrum: Each source sets up a field in space, and then objects respond to the field present right at their locations.
Maxwell put mathematical muscle behind Faraday’s field concept (c 1865), and used his new equations of electrodynamics to make the startling conclusion that light is an electromagnetic phenomenon. Experiments by Hertz about 20 years later strongly supported this hypothesis, and by now the evidence piled up in a century and a half is overwhelming.
But it wasn’t until about 1900, when vectors came into common use, that mathematicians created a gadget called a vector field. Roughly speaking, a vector field is the assignment of an arrow at each point in space. Mathematical vector fields are the current state of the art mathematical models for physical force fields; at each point in space, the direction of the modelling arrow indicates the direction of the force field, and the length of the arrow indicates the strength of the physical field.
You can make a connection between the lines of force favoured by Faraday and our current understanding of vector fields by imagining a tiny car moving along each line of force. At each point in space, the beam of the car’s headlights point in the direction of the vector field arrow at that point. (For all you calculus lovers, we would say that at each point on the line of force, the corresponding vector field arrow is tangent to the line of force.) Or you can imagine that each iron filing in the magnetic field pattern is a little arrow.
It’s fashionable nowadays to eschew mechanical pictures/models of microscopic physics, such as tentacles under “tension” to describe such things as forces. It’s much more popular to take a more abstract approach, and to think of a field in terms of its mathematical model: as the assignment of an arrow (which amounts to a collection of numbers if you think of a vector as list of components) at each point of space. However, it’s worth considering that it was Faraday’s intuition that came first, and Maxwell’s equations came later. That is, creative scientists often think in terms of images of some sort (Einstein reported that he often operated with kinesthetic senses); once they have created a useful idea, they often then express the idea more abstractly, mathematically, and often never report their original images. Schools tend to teach the finished, mathematical product, emphasizing the logical connections between the new idea and the existing scientific complex of ideas. The generative aspect of the idea is almost never discussed in school … unfortunately, this means that not only are high-school and undergraduate students typically not trained to be creative, but they do not even become aware of the creative process in science either.
Poincare said that, “Science is no more a collection of facts than a house is a heap of stones.” Unfortunately, mathematics and science are typically taught in schools as if they were just heaps of stones.
End of rant, and back to our story.
The field perspective gives one a quite dynamic picture of space. Although space may be empty of air far from the earth, nevertheless it is roiling with all kinds of stuff, and so is hardly a vacuum: fields of all kinds are present.
In very recent times (starting in the 1930s), with the development of quantum field theory, our understanding of all fundamental particles and interactions is in terms of fields. Every fundamental particle (electron, quark, neutrino, etc.) is considered to be an excitation (a “quantum”) of a certain type of field. So besides the “force” fields (electric, magnetic, gravitational, nuclear (strong and weak)), we also have “matter” fields.
Einstein’s theory of special relativity is both a theory and a meta-theory; the latter aspect means that it specifies restrictions of various kinds that all physical theories must satisfy. One of the restrictions is that information should not be transmitted (in any way whatsoever) at a speed greater than a certain limiting speed, which happens to be the speed of light. The field concept is just the thing needed to make sure that this restriction is implemented in physical theories.
For example, suppose that the sun were to suddenly change its position; in the action-at-a-distance approach, there is no natural way to explain why there should be a time delay in the transmission of the effect to a distant object, such as earth. In the field approach, there is a natural way: the motion of the sun would create ripples in the field, which would move with a characteristic speed. Only when the ripples reached earth would the earth “notice” that there had been a change.
Ripples in electromagnetic fields have been created, observed, measured, studied, and understood in tremendous detail. These ripples are called electromagnetic waves, which include visible light, x-rays, microwaves, radio waves, and so on.
However, gravitational waves have not yet been observed, as they are expected to be extremely weak. Devices have been constructed to attempt their observation, but no luck yet. Nevertheless, the field concept has been very fruitful in describing gravitational phenomena as well.
A final note: When I was a child, there was a science fiction TV program called Lost in Space. Practically every episode of this program featured what they called “force fields,” but which have nothing to do with the technical meaning of the word as currently understood in modern physics (and as I’ve tried to describe here). Rather, what the TV program called a force field manifested as an invisible barrier. I recall the actors in the program walking along and then suddenly recoiling when they encountered one of these invisible barriers, then exclaiming, “We’ve just run into a force field!” If you’ve run into the science-fiction force field, note that it does not correspond to our current picture of fields in physics or mathematics.
Lost in Space is the original source of the phrase, “Danger Will Robinson!!” Apparently this phrase is popular in computer hacker circles, but I have only seen it in the excellent LaTeX manual, The Not So Short Introduction to LaTeX 2ε, by Tobias Oetiker et al.
(This post first appeared at my other (now deleted) blog, and was transferred to this blog on 25 January 2021.)
